Calculus 2, part 1 of 2: Integrals with applications

A total of 56 hours of lectures

This is an academic level course for university and college engineering. Due to its size, it is divided into two parts. This page describes the first of those two parts.

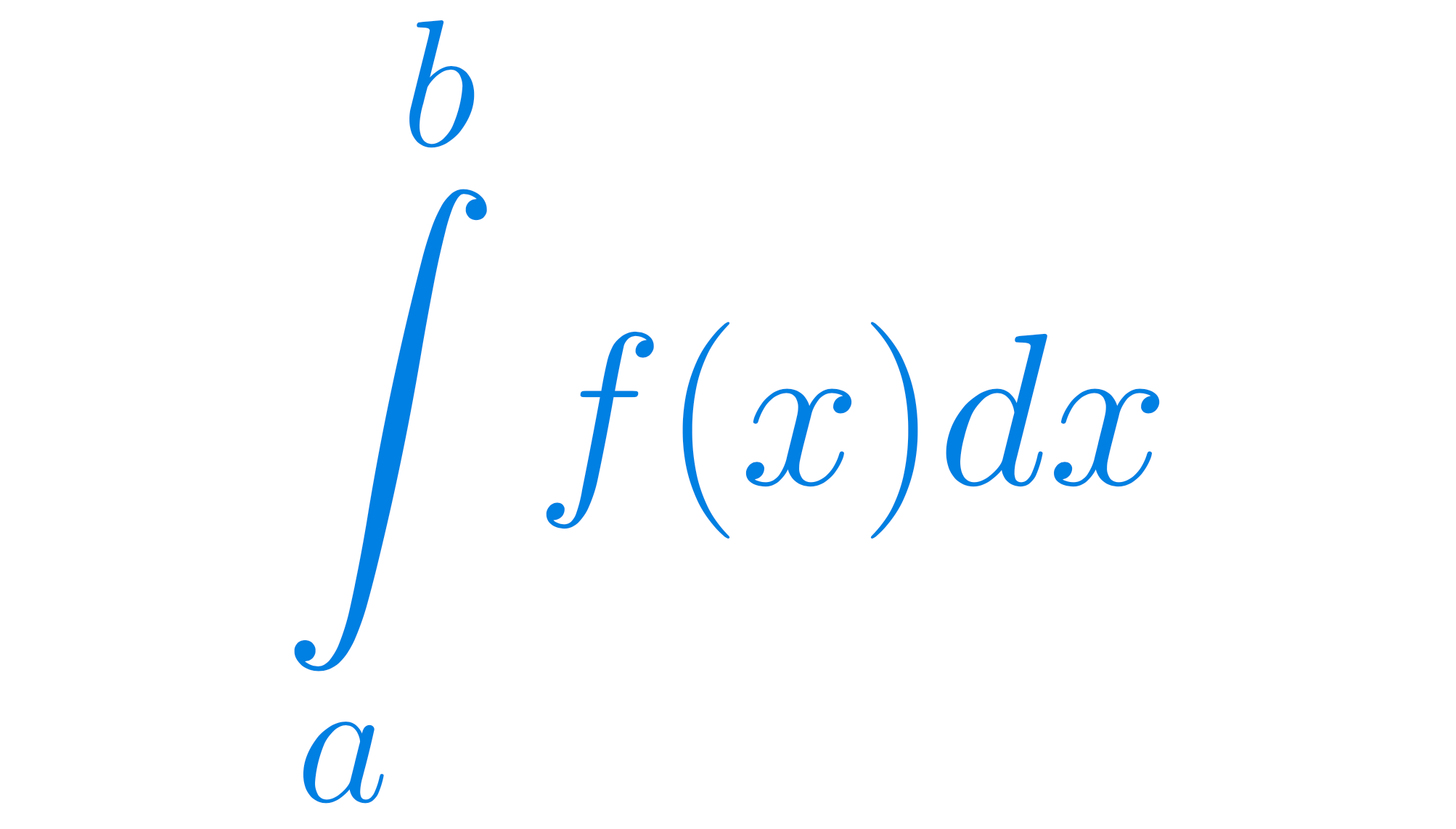
Level - Intermediate

You need to be familiar with the contents of the four Precalculus courses and Calculus 1, part 1 and 2 to comfortably follow along in this course.

Curriculum

Make sure that you check with your professor what parts of the course you will need for your exam. Such things vary from country to country, from university to university, and they can even vary from year to year at the same university.

Calculus 2, part 1 of 2

h

Get the outline

A detailed list of all the lectures in part 1 of the course, including which theorems will be discussed and which problems will be solved. If you are looking for a particular kind of problem or a particular concept, this is where you should look first.

Get Calculus 2 part 1 on Udemy

When you buy the course on Udemy, you get access to it for life. There is just a one-time fee. The prices do vary a lot on Udemy, but if you use our link by clicking on this panel, you will get the best current price. See also our page on “coupon codes” in the menu (the current code is TPOT_APR25).

Course Objectives & Outcomes for part 1

Z
How to solve problems concerning integrals of real-valued functions of 1 variable (illustrated with 419 solved problems) and why these methods work.
Z
Integration by parts as the Product Rule in reverse with many examples of its applications.
Z
Integration of rational functions with help of partial fraction decomposition.
Z
Direct and inverse substitutions; various types of trigonometric substitutions.
Z
Euler’s substitutions.
Z
Riemann integral (definite integral): its definition and geometrical interpretation in terms of area.
Z
Oscillatory sums; Cauchy criterion of (Riemann) integrability.
Z
Proof of uniform continuity of continuous functions on a closed bounded interval.
Z
Integration by inspection: Riemann integrals of odd (or: even) functions over compact and symmetric-to-zero intervals.
Z
Fundamental Theorem of Calculus (FTC) in two parts, with a proof.
Z
Application of FTC for computing derivatives of functions defined with help of Riemann integrals with variable (one or both) limits of integration.
Z
The Mean-Value Theorem for integrals with proof and with a geometrical interpretation; the concept of a mean value of a function on an interval.
Z
Applications of Riemann integrals: rotational volume.
Z
Applications of Riemann integrals: curve length.
Z
Improper integrals of the second kind (integration of unbounded functions).
Z
The concept of antiderivative / primitive function / indefinite integral of a function, and computing such integrals in a process reverse to differentiation.
Z
Integration by substitution as the Chain Rule in reverse with many examples of its applications.
Z
Various types of trigonometric integrals and how to handle them correctly.
Z
The tangent half-angle substitution (universal trigonometric substitution).
Z
Triangle substitutions.
Z
An example of a function that is not Riemann integrable (the characteristic function of the set Q, restricted to [0,1]).
Z
Sequential characterisation of (Riemann) integrability.

Z
Integrability of continuous functions on closed intervals.
Z
Integration by inspection: evaluating some definite integrals with help of areas known from geometry.
Z
Applications of Fundamental Theorem of Calculus in Calc 2 and Calc3.
Z
Application of FTC for computing limits of sequences that can be interpreted as Riemann sums for some integrable functions.
Z
Applications of Riemann integrals: (signed) area between graphs of functions and the x-axis, area between curves defined by two continuous functions.
Z
Applications of Riemann integrals: rotational area.
Z
Improper integrals of the first kind (integration over an unbounded interval).
Z
Comparison criteria for determining whether an improper integral is convergent or not.

Yusuf Amod E.

Udemy student
Explanation is very simple but effective and very graphical slides.

Josa191

Udemy student

I listened to 50% of the lecture and that’s enough for me to write a review, but of course I will listen to the end 🙂

I have to admit that I am glad that I enrolled in the integral course and it exceeded my expectations. Much more than I expected! I will write in several installments what I recognized in that course:

1.) Every time I ask questions, Dr. Hania really tries to answer every question and never leaves a blank. Not only does she answer questions, but she answers in detail in the fine print. She explains very well and with quality, and she kept emphasizing that if I didn’t understand the answer, I could ask again. I can say that I am delighted with it 🙂 […]

This is a very long and detailed review in 9 points. Too long for our site layout, but I recommend you read it in its entirety on Udemy.com

Frederick S.

Udemy student

Yet another superb course. A bargain at any price, but with the discount coupon from Dr. Wehlou’s website, this is an incredible value for the money. The production quality, graphics, and sound are all just so good. The subject material has been carefully organized and is expertly taught. I learned some new integration techniques and this course had the best explanation of the fundamental theorem of calculus I’ve ever seen. Can’t wait until the next course comes out.

Wanda W.

Udemy student

Lecture is well presented and easy to understand

Richard B.

Lecturer

This is the technical core of the thing come to be called Calculus, to the uninitiated surely a dark maize. But Hania shines the light for the wary wanderer, always showing the next turn, always leading the way. With the fog of doubt gone, the devil of detail quickly gets conquered, and the menacing jungle turns flower garden, every plant in due place. This said, the task in question is more like bike riding than flower picking, practice is paramount, and it then only is for the biker to be to bravely, thoroughly, patiently and conscientiously, endure the pain and the pleasure from each day honestly performing dozens and dozens of the zillion expertly crafted exercises.

Andrzej P.

Udemy student

Great course. I love how many optional details are included, in case the students want to understand the topics in more depth. Also, there are so many solved exercises!